Properties of ATOM.
Nuclear properties:~
About 339 nuclides occur naturally on Earth, of which 254 (about 75%) have not been observed to decay, and are referred to as "stable isotopes". However, only 90 of these nuclides are stable to all decay, even in theory. Another 164 (bringing the total to 254) have not been observed to decay, even though in theory it is energetically possible. These are also formally classified as "stable". An additional 34 radioactive nuclides have half-lives longer than 80 million years, and are long-lived enough to be present from the birth of the solar system. This collection of 288 nuclides are known as primordial nuclides. Finally, an additional 51 short-lived nuclides are known to occur naturally, as daughter products of primordial nuclide decay (such as radium from uranium), or else as products of natural energetic processes on Earth, such as cosmic ray bombardment (for example, carbon-14).
For 80 of the chemical elements, at least one stable isotope exists. As a rule, there is only a handful of stable isotopes for each of these elements, the average being 3.2 stable isotopes per element. Twenty-six elements have only a single stable isotope, while the largest number of stable isotopes observed for any element is ten, for the element tin. Elements 43, 61, and all elements numbered 83 or higher have no stable isotopes.
Stability of isotopes is affected by the ratio of protons to neutrons, and also by the presence of certain "magic numbers" of neutrons or protons that represent closed and filled quantum shells. These quantum shells correspond to a set of energy levels within the shell model of the nucleus; filled shells, such as the filled shell of 50 protons for tin, confers unusual stability on the nuclide. Of the 254 known stable nuclides, only four have both an odd number of protons and odd number of neutrons: hydrogen-2 (deuterium), lithium-6, boron-10 and nitrogen-14. Also, only four naturally occurring, radioactive odd–odd nuclides have a half-life over a billion years: potassium-40, vanadium-50, lanthanum-138 and tantalum-180m. Most odd–odd nuclei are highly unstable with respect to beta decay, because the decay products are even–even, and are therefore more strongly bound, due to nuclear pairing effects.
Mass.
The actual mass of an atom at rest is often expressed using the unified atomic mass unit (u), also called dalton (Da). This unit is defined as a twelfth of the mass of a free neutral atom of carbon-12, which is approximately 1.66×10−27 kg. Hydrogen-1 (the lightest isotope of hydrogen which is also the nuclide with the lowest mass) has an atomic weight of 1.007825 u. The value of this number is called the atomic mass. A given atom has an atomic mass approximately equal (within 1%) to its mass number times the atomic mass unit (for example the mass of a nitrogen-14 is roughly 14 u). However, this number will not be exactly an integer except in the case of carbon-12 (see below). The heaviest stable atom is lead-208, with a mass of 207.9766521 u.
As even the most massive atoms are far too light to work with directly, chemists instead use the unit of moles. One mole of atoms of any element always has the same number of atoms (about 6.022×1023). This number was chosen so that if an element has an atomic mass of 1 u, a mole of atoms of that element has a mass close to one gram. Because of the definition of the unified atomic mass unit, each carbon-12 atom has an atomic mass of exactly 12 u, and so a mole of carbon-12 atoms weighs exactly 0.012 kg.
Shape and size:~
When subjected to external forces, like electrical fields, the shape of an atom may deviate from spherical symmetry. The deformation depends on the field magnitude and the orbital type of outer shell electrons, as shown by group-theoretical considerations. Aspherical deviations might be elicited for instance in crystals, where large crystal-electrical fields may occur at low-symmetry lattice sites. Significant ellipsoidal deformations have recently been shown to occur for sulfur ions and chalcogen ions in pyrite-type compounds.
Atomic dimensions are thousands of times smaller than the wavelengths of light (400–700 nm) so they cannot be viewed using an optical microscope. However, individual atoms can be observed using a scanning tunneling microscope. To visualize the minuteness of the atom, consider that a typical human hair is about 1 million carbon atoms in width. A single drop of water contains about 2 sextillion (2×1021) atoms of oxygen, and twice the number of hydrogen atoms. A single carat diamond with a mass of 2×10−4 kg contains about 10 sextillion (1022) atoms of carbon. If an apple were magnified to the size of the Earth, then the atoms in the apple would be approximately the size of the original apple.
Radioactive decay.
 |
This diagram shows the half-life (T½) of various isotopes with Z protons and N neutrons. |
- Alpha decay: this process is caused when the nucleus emits an alpha particle, which is a helium nucleus consisting of two protons and two neutrons. The result of the emission is a new element with a lower atomic number.
- Beta decay (and electron capture): these processes are regulated by the weak force, and result from a transformation of a neutron into a proton, or a proton into a neutron. The neutron to proton transition is accompanied by the emission of an electron and an antineutrino, while proton to neutron transition (except in electron capture) causes the emission of a positron and a neutrino. The electron or positron emissions are called beta particles. Beta decay either increases or decreases the atomic number of the nucleus by one. Electron capture is more common than positron emission, because it requires less energy. In this type of decay, an electron is absorbed by the nucleus, rather than a positron emitted from the nucleus. A neutrino is still emitted in this process, and a proton changes to a neutron.
- Gamma decay: this process results from a change in the energy level of the nucleus to a lower state, resulting in the emission of electromagnetic radiation. The excited state of a nucleus which results in gamma emission usually occurs following the emission of an alpha or a beta particle. Thus, gamma decay usually follows alpha or beta decay.
Each radioactive isotope has a characteristic decay time period—the half-life—that is determined by the amount of time needed for half of a sample to decay. This is an exponential decay process that steadily decreases the proportion of the remaining isotope by 50% every half-life. Hence after two half-lives have passed only 25% of the isotope is present, and so forth.
Magnetic moment.
The magnetic field produced by an atom—its magnetic moment—is determined by these various forms of angular momentum, just as a rotating charged object classically produces a magnetic field. However, the most dominant contribution comes from electron spin. Due to the nature of electrons to obey the Pauli exclusion principle, in which no two electrons may be found in the same quantum state, bound electrons pair up with each other, with one member of each pair in a spin up state and the other in the opposite, spin down state. Thus these spins cancel each other out, reducing the total magnetic dipole moment to zero in some atoms with even number of electrons.
In ferromagnetic elements such as iron, cobalt and nickel, an odd number of electrons leads to an unpaired electron and a net overall magnetic moment. The orbitals of neighboring atoms overlap and a lower energy state is achieved when the spins of unpaired electrons are aligned with each other, a spontaneous process known as an exchange interaction. When the magnetic moments of ferromagnetic atoms are lined up, the material can produce a measurable macroscopic field. Paramagnetic materials have atoms with magnetic moments that line up in random directions when no magnetic field is present, but the magnetic moments of the individual atoms line up in the presence of a field.
The nucleus of an atom will have no spin when it has even numbers of both neutrons and protons, but for other cases of odd numbers, the nucleus may have a spin. Normally nuclei with spin are aligned in random directions because of thermal equilibrium. However, for certain elements (such as xenon-129) it is possible to polarize a significant proportion of the nuclear spin states so that they are aligned in the same direction—a condition called hyperpolarization. This has important applications in magnetic resonance imaging.
Energy levels.
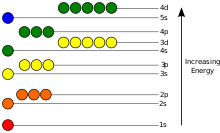 |
These electron's energy levels (not to scale) are sufficient for ground states of atoms up to cadmium (5s2 4d10) inclusively. Do not forget that even the top of the diagram is lower than an unbound electron state. |
The potential energy of an electron in an atom is negative, its dependence of its position reaches the minimum (the most absolute value) inside the nucleus, and vanishes when the distance from the nucleus goes to infinity, roughly in an inverse proportion to the distance. In the quantum-mechanical model, a bound electron can only occupy a set of states centered on the nucleus, and each state corresponds to a specific energy level; see time-independent Schrödinger equation for theoretical explanation. An energy level can be measured by the amount of energy needed to unbind the electron from the atom, and is usually given in units of electronvolts (eV). The lowest energy state of a bound electron is called the ground state, i.e. stationary state, while an electron transition to a higher level results in an excited state. The electron's energy raises when n increases because the (average) distance to the nucleus increases. Dependence of the energy on ℓ is caused not by electrostatic potential of the nucleus, but by interaction between electrons.
For an electron to transition between two different states, e.g. grounded state to first excited level (ionization), it must absorb or emit a photon at an energy matching the difference in the potential energy of those levels, according to Niels Bohr model, what can be precisely calculated by the Schrödinger equation. Electrons jump between orbitals in a particle-like fashion. For example, if a single photon strikes the electrons, only a single electron changes states in response to the photon; see Electron properties.
The energy of an emitted photon is proportional to its frequency, so these specific energy levels appear as distinct bands in the electromagnetic spectrum. Each element has a characteristic spectrum that can depend on the nuclear charge, subshells filled by electrons, the electromagnetic interactions between the electrons and other factors.
 |
An example of absorption lines in a spectrum |
When a continuous spectrum of energy is passed through a gas or plasma, some of the photons are absorbed by atoms, causing electrons to change their energy level. Those excited electrons that remain bound to their atom spontaneously emit this energy as a photon, traveling in a random direction, and so drop back to lower energy levels. Thus the atoms behave like a filter that forms a series of dark absorption bands in the energy output. (An observer viewing the atoms from a view that does not include the continuous spectrum in the background, instead sees a series of emission lines from the photons emitted by the atoms.) Spectroscopic measurements of the strength and width of atomic spectral lines allow the composition and physical properties of a substance to be determined.
Close examination of the spectral lines reveals that some display a fine structure splitting. This occurs because of spin–orbit coupling, which is an interaction between the spin and motion of the outermost electron. When an atom is in an external magnetic field, spectral lines become split into three or more components; a phenomenon called the Zeeman effect. This is caused by the interaction of the magnetic field with the magnetic moment of the atom and its electrons. Some atoms can have multiple electron configurations with the same energy level, which thus appear as a single spectral line. The interaction of the magnetic field with the atom shifts these electron configurations to slightly different energy levels, resulting in multiple spectral lines. The presence of an external electric field can cause a comparable splitting and shifting of spectral lines by modifying the electron energy levels, a phenomenon called the Stark effect.
If a bound electron is in an excited state, an interacting photon with the proper energy can cause stimulated emission of a photon with a matching energy level. For this to occur, the electron must drop to a lower energy state that has an energy difference matching the energy of the interacting photon. The emitted photon and the interacting photon then move off in parallel and with matching phases. That is, the wave patterns of the two photons are synchronized. This physical property is used to make lasers, which can emit a coherent beam of light energy in a narrow frequency band.
Valence and bonding behavior.
The chemical elements are often displayed in a periodic table that is laid out to display recurring chemical properties, and elements with the same number of valence electrons form a group that is aligned in the same column of the table. (The horizontal rows correspond to the filling of a quantum shell of electrons.) The elements at the far right of the table have their outer shell completely filled with electrons, which results in chemically inert elements known as the noble gases.
States.
 |
Snapshots illustrating the formation of a Bose–Einstein condensate |
At temperatures close to absolute zero, atoms can form a Bose–Einstein condensate, at which point quantum mechanical effects, which are normally only observed at the atomic scale, become apparent on a macroscopic scale. This super-cooled collection of atoms then behaves as a single super atom, which may allow fundamental checks of quantum mechanical behavior.



No comments:
Post a Comment